So, you want to build a loudspeaker…
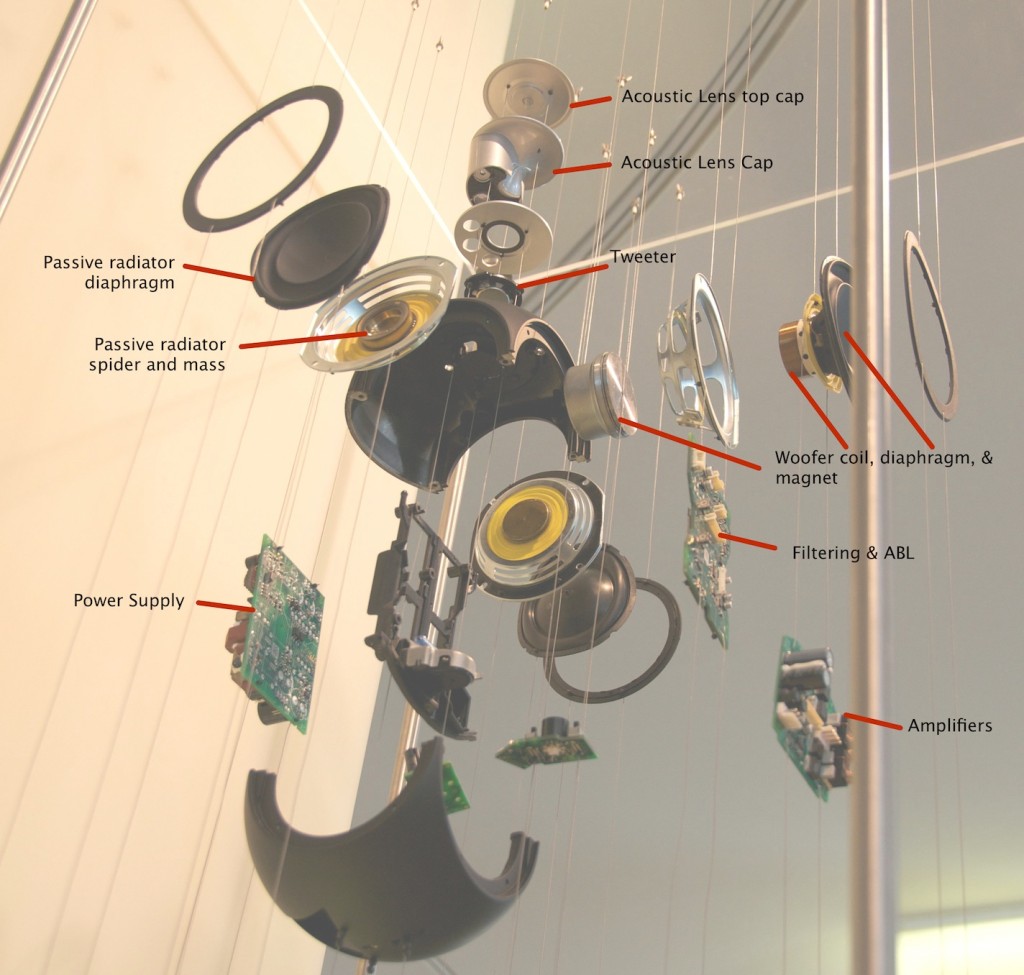
One of the first things you’ll find out is that, if you’re building a loudspeaker with moving coil drivers, and unless you want a loudspeaker with very limited capabilities, you’ll probably need to use more than one driver. Starting small, you’ll at least need a bigger driver to produce the lower frequencies and a smaller driver to produce the higher ones. No surprise so far – many people lead meaningful lives with just a tweeter and a woofer.
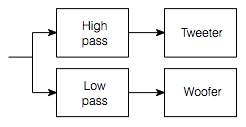
However, you’ll probably need to ensure that the tweeter doesn’t get too much signal at low frequencies, and the woofer doesn’t get too many highs. In order to do this, you’ll need a crossover. Still no surprises. Most people who build a loudspeaker already know that they’ll need a crossover to keep their drivers happier.
Now for some new stuff – at least for some people. When you make a crossover, you must remember to keep the driver’s characteristics in mind. You can’t just slap a high pass filter on the tweeter and a low pass filter on the woofer and expect things to work. The tweeter is already behaving as a high pass filter all by itself. If the characteristics of the tweeter’s inherent high pass are what you want, then you don’t want to duplicate that filter in the electronics. So, design your filters wisely. I will probably come back to some examples of this some time in a future posting.
However, that is not the topic for today. For today, we will assume that we are building a loudspeaker using two very special drivers. They are:
- infinitely small
- have bandwidths that go from DC to infinity
- have “perfect” impulse responses
- and therefore have completely flat phase responses
In other words, we will pretend that each of our drivers is a perfect point source. We’ll also assume that they are not mounted on a baffle (a fancy way of saying “on the front of a box” – usually…). Instead, they’re just floating in space, arbitrarily 25 cm apart (one directly above the other). We’ll arbitrarily make the crossover frequency 500 Hz. Finally, let’s say that we’re arbitrarily 2 m away from the loudspeaker.
The reason for all of these assumptions is that, for the purposes of this posting, we’re only interested in the effects of the crossover on the signal, so I’m making everything else in the system either perfect or non-existent. Of course, this has nothing to do with the real world, but I don’t really care today.
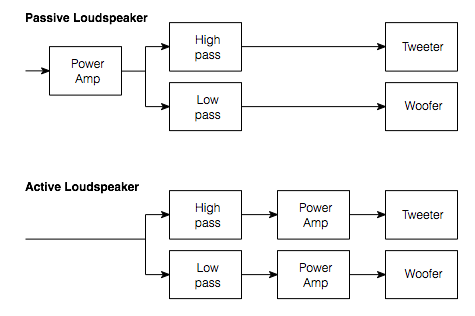
So, if you’ve done a little research, you’ll know that there are a plethora of options to chose from when it comes to crossovers. I’ll assume that we’re building an active loudspeaker with a DSP so we can do whatever we want.
Linkwitz Riley, 4th Order
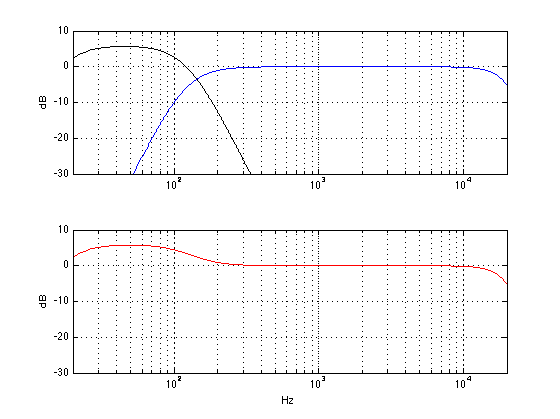
Let’s start with Old Faithful: a 4th-order Linkwitz-Riley crossover. This is implemented by putting two 12 dB/oct Butterworth filters in series, each with a cutoff frequency equal to the intended crossover frequency. (If you’re using biquads, set your Q to 1/sqrt(2) on each filter). The total low pass section will have a gain of -6.02 dB at the crossover frequency (so will the total high pass section). Since the two sections are 360° out of phase with each other at all frequencies, they’ll add up to give you a total of 0 dB when they sum together at any frequency. However, you must remember that the filter sections used in the crossover have an effect on the phase response of the re-combined signal. As a result, when the two are added back together (at the listening position) the total will also have a modified phase response – even when you are on-axis to the loudspeakers, (and equidistant to the two loudspeaker drivers).
It is also important to remember that the phase relationship of the two sections (coming from the tweeter and the woofer) is only correct when those two drivers are the same distance from the listener. If the tweeter is a little closer to you (say, because the tweeter is on top and you stood up) then its signal will arrive too early relative to the woofer’s and the phase relationship of the two signals will be screwed up, resulting in an incorrect summing of the two signals.
How much the total is screwed up depends on a bunch of factors including
- the relative phase responses of the filters in the crossover
- the phase responses of the drivers (we’re assuming for this posting that this is not an issue, remember?)
- the deviation in those phase responses caused by the mis-alignment of distances to the drivers
The result of this is a deviation in the vertical off-axis response of the loudspeaker. How bad is this? Let’s look!
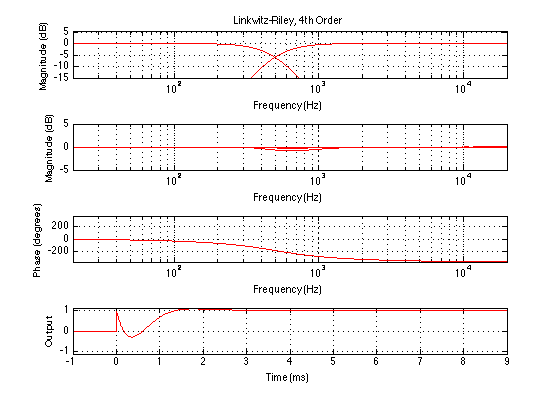
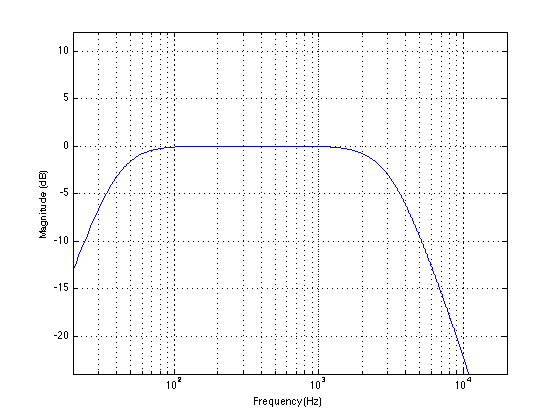
This figure shows 4 plots. The top one shows the magnitude responses of the two individual sections. As you can see, the crossover frequency is 1 kHz, and both sections are 6 dB down at that frequency.
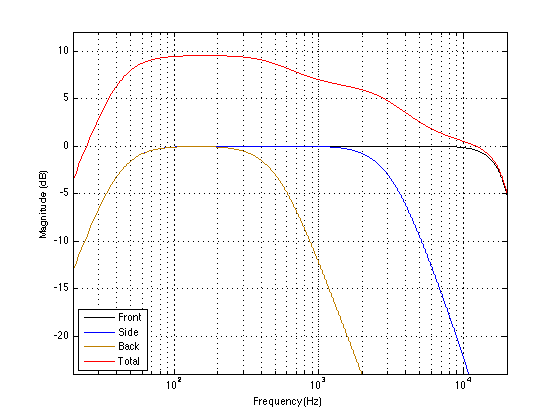
The second plot shows the total magnitude responses at 5 different vertical angles of incidence to the loudspeaker: -30°, -15°, 0°, 15°, and 30°. So, we’re going from below the loudspeaker to above the loudspeaker. It’s not obvious which plot is for which angle because, for the purposes of this discussion, it doesn’t matter. I’m only interested in talking about how different the loudspeaker sounds at different angles – not the specifics of how it sounds different.
The third plot shows the total phase response of the system, at a position that is on axis to the loudspeaker (and therefore equidistant to both drivers). As you can see there, a perfect 2-way loudspeaker with a 4th order Linkwitz-Riley crossover behaves as a 4th-order allpass filter. In other words, at low frequencies, the output is in phase with the input. At the crossover frequency, the output is 180° out of phase with the input. At high frequencies, the output is 360° out of phase with the input.
The fourth plot shows the step response of the total system, at a position that is on axis to the loudspeaker (and therefore equidistant to both drivers). As you can see there, a perfect 2-way loudspeaker with a 4th order Linkwitz-Riley crossover does not give you a “perfect” step response – it can’t, since it acts an allpass filter. The weird shape you see there is cause by the fact that the high frequencies are not “in phase” with the low frequencies. (I know, I know… different frequencies cannot be “in phase”.) Since different frequencies are delayed differently by the total system, they do not add up correctly in the time domain. Thus, although the total output in terms of magnitude is flat (hence the flat on-axis frrequency response) the time response will be weird.
Looking in detail at the step response plot, you can see that it takes about 1.5 ms for the total output to settle to a value of 1. The actual time that it takes is dependent on the crossover frequency. The lower the frequency, the longer it will take. It’s the shape of the step response that’s determined by the crossover’s phase response. What can be seen from the shape is that the high-frequency spike hits first (as we would expect), then the step response drops back to a negative value before heading upwards. It overshoots, peaking at a value of 1.0558 before coming back down, undershooting slightly (to a value of 0.9976) and finally settling at a value of 1. Note that these values won’t change with changes in crossover frequency – they’ll just happen at a different time. The higher the frequency, the faster the response.
Whether or not this modified time response is worth worrying about (i.e. can you hear it) is also outside of the scope of today’s discussion. All we’re going to say for today is that this temporal distortion exists, and it is different for different crossover strategies as we’ll see below.
Linkwitz-Riley 2nd Order
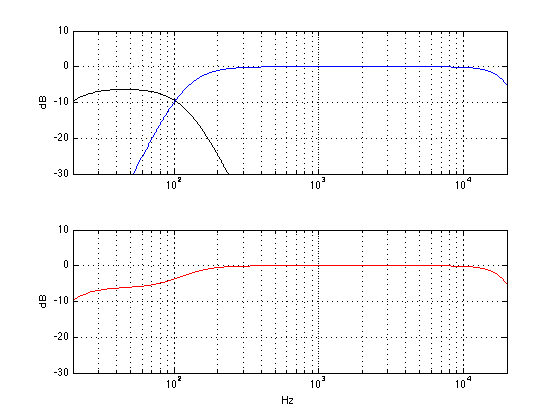
A second possible crossover strategy is to use a 2nd-order Linkwitz Riley. This is similar to a 4th-order, except that instead of putting two 12 dB/octave Butterworth filters in series to make each section, you put two 6 dB/octave Butterworth filters in series.
Since the total filters applied to make the high pass and low pass sections of this crossover are each made with only two first-order filters (instead of two second-order filters), the high pass and low pass sections are only 180 degrees out of phase with each other (at all frequencies). Consequently, in order to get them to add back together without cancelling completely at the crossover frequency, you have to invert the polarity of one of the sections. (We’ll do this to the high pass section, just in case you can hear your woofers pulling when they ought to push when a kick drum hits). On the plus side, since they’re 180 degrees out of phase at all frequencies, if you DO flip the polarity of your high pass section, they’ll add back together (on axis) to give you a flat magnitude response.

As you can see in the above plots, the slopes of the high pass and low pass sections in this crossover type are more gentle than in the 4th order Linkwitz Riley. This should be obvious, since they have a lower order. In the second plot, you can see that, on-axis, the magnitude response is flat, just as we would expect. However, there are implications on the off-axis response. The deviation from “flat” is greater with the 2nd-order LR than it is with the 4th-order version. Not by much, admittedly, but it is greater. So, if you’re concerned about deviations in your off-axis response in the vertical plane, you might prefer the 4th-order LR over the 2nd-order variant.
If, however, you lay awake at night worring about phase response (you know who you are – yes – I’m talking you YOU) then you might prefer the 2nd-order Linkwitz Riley, since, as you can see in the third plot, the total output is only 180° out of phase with its input in the worst case – only half that of the 4th-order variant. On the other hand, since it’s 180° out of phase, that means that a high voltage going into the system (at high frequencies) will come out as a low pressure. So, if you’re the kind of person who lays awake at night worrying about “absolute phase” (you know who you are – yes – I’m talking to YOU) then this might not be your first choice.
Finally, take a look at the step response in the final plot. You’ll notice immediately that the high frequencies are 180° out iof phase, since the initial transient of the step goes down instead of up. You’ll also notice that the step “recovers” to a value of 1 a little faster than the 4th order Linkwitz Riley. Note that, a 2nd order LR, doesn’t have the overshoot that we saw in the 4th order version.
Butterworth, 12 db/octave
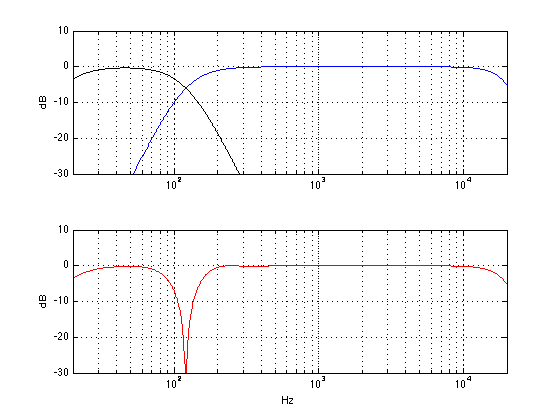
Possibly the most common passive crossover type (and therefore, possibly the most common crossover type, period!) is the 12 dB/octave Butterworth crossover. This is made by using a 2nd-order Butterworth filter for each section (the high pass and the low pass).

You’ll notice in the top plot that this means that the filter sections are only 3 dB down at the crossover frequency. This has some implications on the on-axis response. Since the two filter sections are 180° out of phase with each other (at all frequencies – just like the 2nd-order LR crossover) then we have to flip the polarity of one of the sections (the high-pass section again, for all the same reasons) to prevent them from cancelling each other at the crossover frequency when they’re added back together. However, now we have a problem. Since the two sections are in-phase (due to the 180° phase shift plus the polarity flip) and since they’re only 3 dB down at the crossover frequency, when they get added back together, you get more out than you put into the system. This can be seen in the second plot, where the total magnitude response has a bump at the crossover frequency – even when on-axis.
Of course, a 3 dB bump in the magnitude response will be audible, at the very least as a change in timbre (3 dB is, after all, twice the power). We can also see that there is a small, but visible change in the overall magnitude response as you change the vertical angle to the listener.
The third plot shows that a 12 dB/Octave Butterworth crossover, when all other issues are ignored, acts as a 2nd-order allpass filter with a worst-case phase distortion of 180°.
Finally, the fourth plot shows that its step response is similar, but not identical to, the 2nd-order LR crossover. The initial transient goes negative because we have inverted the polarity of the high pass section. Unlike the 2nd-order LR (but similar to the 4th-order LR), however, there is an overshoot and undershoot before the response settles at a value of 1. That overshoot reaches a maximum of 1.1340, and the subsequent undershoot goes down to 0.9942.
Butterworth, 18 db/oct
Sometimes, you’ll also hear of people using an 18 dB/octave Butterworth crossover instead of the 12 dB/octave version. These are a little more complicated to implement, but not uncommon.
The responses of this crossover type are plotted below.
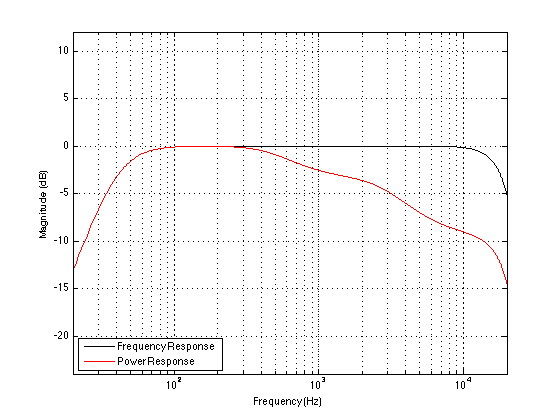
The top plot shows that, although the order of the Butterworth high pass and low pass sections are higher (and therefore have steeper slopes), they are still only 3 dB down at the crossover frequency. However, since the two sections are now 270° out of phase, they add together to give you a magnitude of 0 dB – the same as the input – but only when you’re on-axis. As can be seen in the second plot, the off-axis response of an 18 dB/Octave Butterworth crossover is MUCH worse than all of the other crossover types we’ve seen so far. So, if you’re the type of person who worries about off-axis response, or the magnitude response of your ceiling and floor reflections, or the power response of your loudspeaker, then you probably wouldn’t choose this crossover over the previous ones.
The phase response of the total output of this crossover seems a bit strange initially, since you have two filters that are 270° apart at all frequencies, but the summed output has the phase response of a 4th-order allpass. However, what is not seen in this plot are the individual phase responses of the two sections. The low pass section has a phase response that starts at 0° in the low end and drops to -270° in the high end. The high pass section’s phase response starts at -90° in the low end and ends at -360° in the high end. So, although the two sections, individually, have phase response curves that have a similar shape to a third-order allpass, their combined outputs result in a 4th order allpass.
Finally, let’s come to the step response. This one is the busiest one yet, since, after the initial transient and drop, it overshoots (to a value of 1.178), then undershoots (0.971), then overshoots again (1.005) and finally undershoots (0.9994) before finally settling at a value of 1.
Constant Voltage, (using a Butterworth, 18 db/oct high pass)
There is a group of persons who believe that the step response (or the shape of a square wave through the system) is the be-all-and-end-all for determining the quality of a system. The logic goes that, if a square wave goes in, and a square wave comes out, then the system is perfect. This is true – if you mean “perfect at reproducing square waves” – which may or may not be important.
Following this logic, the idea is that, if you take your initial input and make a filtered version, then all you need to do is to subtract that filtered version from the input to get the remainder. If you then add the remainder and the filtered section, you get out what you put in, so the system is perfect. At least, that’s the idea. Let’s see how well that works out, shall we?
(By the way, the name for this classification of crossovers is “constant voltage” crossovers, and there are lots of different ways to implement them. Richard Small wrote some good stuff about them in some AES papers once-upon-a-time, if you’re curious.)
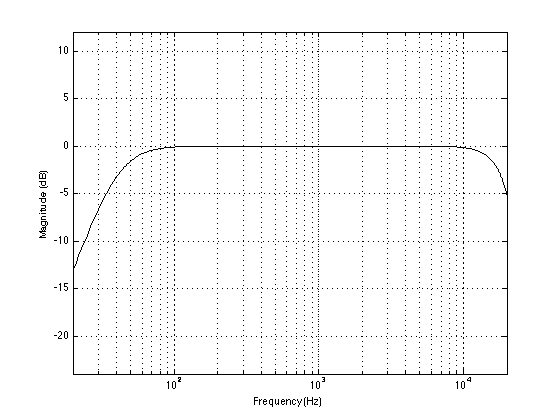
So, let’s look at one fairly-common implementation of a constant voltage crossover. We’ll take the input, filter it with an 18 dB / Octave Butterworth filter and use that for the high pass section. The low pass section is created by subtracting the high pass section from the input, and we just take what we get.
As you can see in the top plot, the result of this is that the low pass section is a little weird. It has a rather large bump in its magnitude response around the crossover frequency. In addition, the slope of the high pass roll-off is not very steep. This all might be okay, if your lower driver is able to handle it, but it might not. (Note that, if we had made a Butterworth low pass and used the subtraction trick to get the high pass section, the bump would have appeared in the high pass section, which would result in too much low-frequency energy in the tweeter, thus likely making it unhappy… That’s why we used a Butterworth high pass to start.)
Let’s skip the next plot and look at the third and fourth. As you can see in both of these, the constant voltage crossover is unique in that it has no phase distortion, and the step response is perfect. This is to be expected, since these are the primary criteria behind the design of this type of crossover.
Now, let’s look at the second plot. As you can see there, the off-axis response of a constant voltage crossover is a complete disaster. So, if you’re the kind of person who thinks that off-axis response is important – or at least worth considering, you should probably stay away from this crossover. However, if you have an acoustically absorptive floor and an acoustically absorptive ceiling (so no vertical reflections) and you never stand up, and you’re inside the room’s critical distance with respect to the loudspeaker, then this little problem might not be an issue for you.
Concluding comments
The thing that you have to remember for all of the stuff I’ve said here is that it’s only applicable within the limitations of the parameters I stated at the beginning. If your drivers are imperfect, or if you have a high pass in series with your low pass section because your loudspeaker driver exists in real life (it does), or if you have a symmetrical driver arrangement (sometimes called a D’Appolito design, named after the first person to be smart enough to publish a paper about it), then all of these results will be different.
Also, the other important thing to remember is that I’m making no claims about whether these “problems” are audible. They might be – and they might not be. But don’t just jump to conclusions all willy-nilly and assume that, because you can see a difference in these plots, you’ll be able to hear the difference in your loudspeakers. Then again, that doesn’t mean that I’m saying “you can’t!” What I’m saying is “I don’t know whether you can hear these issues or not – any of them.”
P.S .
Happy New Year.